Factors of 20
When it comes to numbers, exploring their factors is an intriguing endeavor. In this article, we will delve into the world of factors, focusing specifically on the factors of 20. We will discuss what factors are, how they relate to multiplication and division, and explore the various divisors of the number 20. By the end of this article, you will have a comprehensive understanding of the factors of 20 and their significance in mathematics. So let’s dive in!
Understanding Factors
Before we explore the factors of 20, let’s first understand what factors actually are. In mathematics, a factor refers to any number that can be evenly divided into another number without leaving a remainder. Put simply, factors are the numbers that multiply together to give us a specific number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12, as these numbers can divide by 12 without leaving a remainder. When you apply any of the explained methods you will get to know the Factors Of 30 accurately.
The Factors of 20
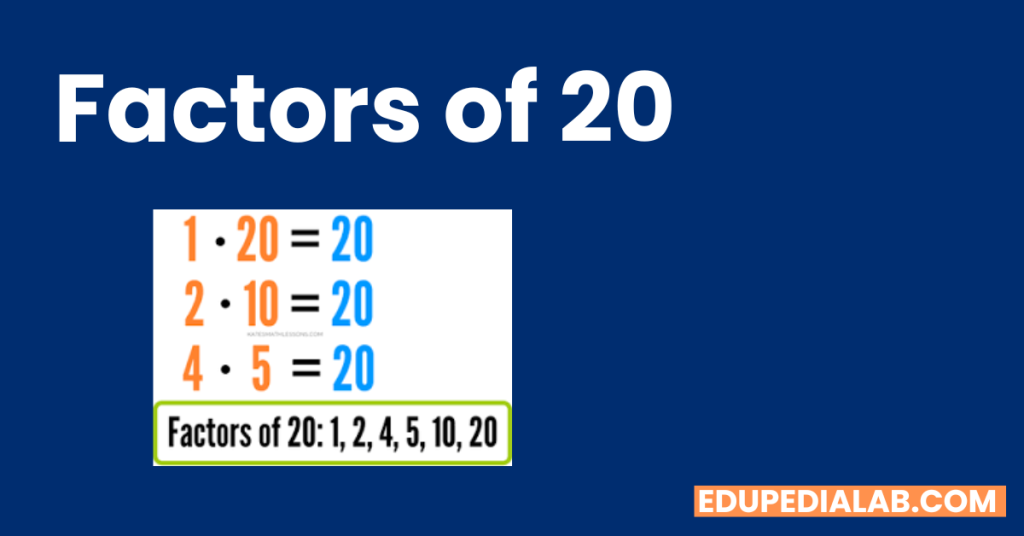
Now that we have a basic understanding of factors, let’s focus on the factors of 20. To determine the factors of 20, we need to find all the numbers that can divide 20 without leaving a remainder. Let’s list them out:
1. The Number 1
The number 1 is a universal factor of all numbers since any number divided by 1 is always itself. Therefore, 1 is a factor of 20.
2. The Number 2
The number 2 is another factor of 20 because 20 divided by 2 equals 10 with no remainder.
3. The Number 4
The number 4 is a factor of 20 since 20 divided by 4 equals 5 without any remainder.
4. The Number 5
The number 5 is a factor of 20 because 20 divided by 5 gives us 4 with no remainder.
5. The Number 10
The number 10 is also a factor of 20 because 20 divided by 10 equals 2 without leaving any remainder.
6. The Number 20
Lastly, the number 20 itself is a factor as any number divided by itself equals 1. Thus, 20 is a factor of 20.
Conclusion
In conclusion, the factors of 20 are 1, 2, 4, 5, 10, and 20. These numbers can be divided evenly into 20 without leaving any remainder. Understanding factors and their role in mathematics allows us to grasp the concept of divisibility and find the divisors of a given number. The factors of 20 help us comprehend the number’s mathematical properties and provide a foundation for further exploration in the field of number theory.
FAQs (Frequently Asked Questions)
- What is a factor?
- A factor is a number that divides another number without leaving a remainder.
- How do you find the factors of a number?
- To find the factors of a number, you need to determine all the numbers that can divide it without leaving a remainder.
- Are 1 and the number itself always factors?
- Yes, 1 and the number itself are always factors of any given number.
- What is the importance of understanding factors?
- Understanding factors helps us comprehend divisibility, find divisors, and analyze the mathematical properties of numbers.
- Can a number have more than one set of factors?
- No, a number can only have one set of factors, but the order in which they are listed may vary.
- What is the greatest factor of 20?
- The greatest factor of 20 is 20 itself.
- How many factors does 20 have?
- The number 20 has six factors: 1, 2, 4, 5, 10, and 20.
- Can the factors of 20 be used to find the factors of other numbers?
- Yes, the factors of 20 can be used as a reference to find the factors of other numbers, particularly those that have 20 as a factor.
- Are the factors of 20 the same as its multiples?
- No, factors are numbers that divide evenly into a given number, while multiples are numbers obtained by multiplying a given number by an integer.
- Are factors only applicable to whole numbers?
- Factors can be applied to any number, including whole numbers, fractions, and even irrational numbers.
Now that we have covered the basics of factors and explored the factors of 20, let’s delve deeper into the significance of factors in mathematics.
Factors play a crucial role in various mathematical operations and concepts. They help us understand the divisibility of numbers and identify common multiples and divisors. By examining the factors of a number, we can analyze its mathematical properties and relationships with other numbers.
In the case of 20, its factors provide valuable insights. For instance, knowing that 20 has factors such as 2 and 10 allows us to determine that it is divisible by 2. Similarly, the factors 4 and 5 indicate that 20 is divisible by 4 and 5 as well.
Factors are particularly useful when dealing with fractions and simplifying them. By identifying common factors between the numerator and denominator, we can simplify fractions and make calculations more manageable.
Furthermore, factors play a role in prime factorization, which involves expressing a number as the product of its prime factors. The prime factorization of 20 is 2 x 2 x 5, where 2 and 5 are the prime factors of 20. This representation helps in various mathematical computations, such as finding the greatest common divisor or simplifying square roots.
In real-world applications, understanding factors is essential in fields such as engineering, finance, and cryptography. Factors are utilized in tasks like prime number generation, data encryption algorithms, and determining optimal solutions for complex problems.
In conclusion, the factors of 20—1, 2, 4, 5, 10, and 20—are integral components of understanding its mathematical properties and relationships. Factors allow us to explore divisibility, simplify fractions, and perform prime factorization, among other mathematical operations. By grasping the concept of factors and their significance, we gain a deeper understanding of the intricacies of numbers and their applications in various fields.