Factors Of 21
When it comes to numbers, they hold a fascinating world of patterns and relationships. One such number is 21. In this article, we will delve into the factors of 21, uncovering the multiplicative components that make up this intriguing number. By understanding its factors, we can gain insight into its properties and explore its significance in various mathematical contexts. So let’s embark on this numerical journey and unravel the factors of 21. You can solve the factors Of 14 on your own if you learn the basics of finding the factors.
Factors Of 21: An Overview
To comprehend the factors of 21, we need to identify the numbers that divide 21 without leaving a remainder. These numbers are called factors, and they play a crucial role in understanding the properties of any given number. The factors of 21 are the numbers that, when multiplied together, result in the product of 21.
The Factors of 21: Exploring the Possibilities
Prime Factorization of 21
To determine the prime factors of 21, we can begin by dividing it by prime numbers. The first prime number to check is 2, but since 21 is an odd number, we move on to the next prime number, 3. Dividing 21 by 3 gives us 7, which is a prime number. Therefore, the prime factorization of 21 is 3 * 7.
Divisors of 21
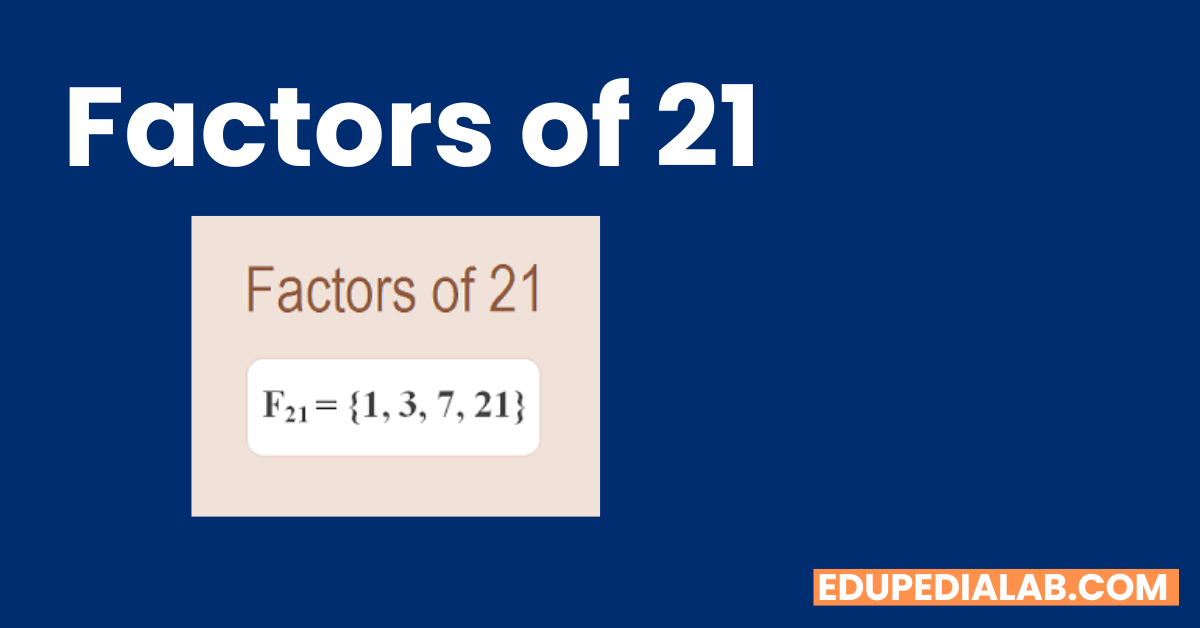
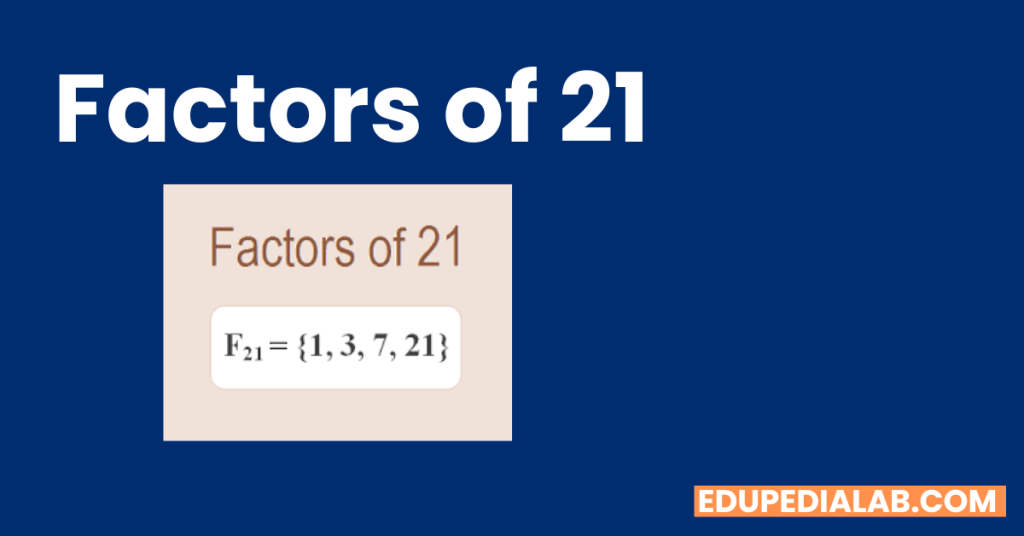
Apart from prime factors, it is also essential to consider all the divisors of 21. Divisors are the numbers that evenly divide a given number. In the case of 21, the divisors are 1, 3, 7, and 21. These numbers, when multiplied in pairs, result in the product of 21.
The Significance of Factors of 21
The factors of 21 play a significant role in various mathematical concepts and applications. Let’s explore a few areas where the factors of 21 have relevance:
- Arithmetic: In arithmetic, the factors of 21 help in simplifying fractions and finding common denominators.
- Algebra: In algebraic equations, the factors of 21 assist in factoring polynomials and solving quadratic equations.
- Number Theory: Factors provide insights into number patterns, divisibility rules, and prime factorization.
- Geometry: The factors of 21 contribute to understanding geometric shapes, such as rectangles and squares, where their sides’ lengths are determined by factors.
- Probability: Factors help calculate probabilities by determining the possible outcomes of an event.
Frequently Asked Questions (FAQs)
FAQ 1: What are the prime factors of 21?
The prime factors of 21 are 3 and 7. These are the prime numbers that, when multiplied together, give us the product of 21.
FAQ 2: How can I find the factors of 21?
To find the factors of 21, you need to identify the numbers that divide 21 without leaving a remainder. These numbers are 1, 3, 7, and 21.
FAQ 3: Are there any other ways to represent 21 as a product of two numbers?
Yes, 21 can also be represented as the product of 1 and 21. However, when talking about factors, we usually exclude 1 and the number itself, as they are considered trivial factors.
FAQ 4: Can the factors of 21 be negative?
No, the factors of 21 are always positive integers. Negative numbers are not considered factors in this context.
FAQ 5: How do the factors of 21 relate to its multiples?
The factors of 21 are intimately connected to its multiples. A multiple of 21 is any number that can be obtained by multiplying 21 by another integer. These multiples can be expressed as the product of 21 and its factors. For example, the first few multiples of 21 are 21, 42, 63, 84, and so on, where each multiple is obtained by multiplying 21 by a different factor.
FAQ 6: Can the factors of 21 be used in real-life applications?
Absolutely! The factors of 21 find applications in various real-life scenarios. For instance, if you have a group of 21 people and you want to divide them into equal teams, the factors of 21 (1, 3, 7, and 21) will help you determine the possible team sizes. Additionally, factors are essential in understanding and solving problems related to division, fractions, and ratios.
Conclusion
In conclusion, the factors of 21 provide valuable insights into the composition and properties of this unique number. By understanding its factors, we can unlock a deeper understanding of various mathematical concepts, such as prime factorization, divisibility, and problem-solving. The factors of 21, including 1, 3, 7, and 21, have practical applications in fields like arithmetic, algebra, geometry, number theory, and probability. Embracing the world of factors allows us to explore the rich tapestry of numbers and their intricate relationships.