Factors Of 17
In the realm of mathematics, factors play a crucial role in understanding the properties and relationships of numbers. Factors are the numbers that divide another number without leaving a remainder. In this article, we will delve into the fascinating world of the factors of 17. By exploring the divisors of 17, we will gain insights into the unique characteristics of this prime number. The number of Factors Of 34 is 4 including 1,2,17 and 34.
1. What is a Prime Number?
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, a prime number cannot be evenly divided by any other number except 1 and the number itself. Prime numbers possess unique properties and have been a subject of fascination for mathematicians throughout history.
2. Understanding Factors
Factors are the numbers that divide another number without leaving a remainder. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12. Factors provide insights into the divisibility and structure of numbers, helping us analyze their properties and relationships.
3. The Number 17
The number 17 is a prime number, meaning it has only two distinct factors: 1 and 17. It falls into the category of numbers that are indivisible by any other number except 1 and itself. Let’s explore the factors of 17 to gain a deeper understanding of this unique prime number.
4. Factors of 17
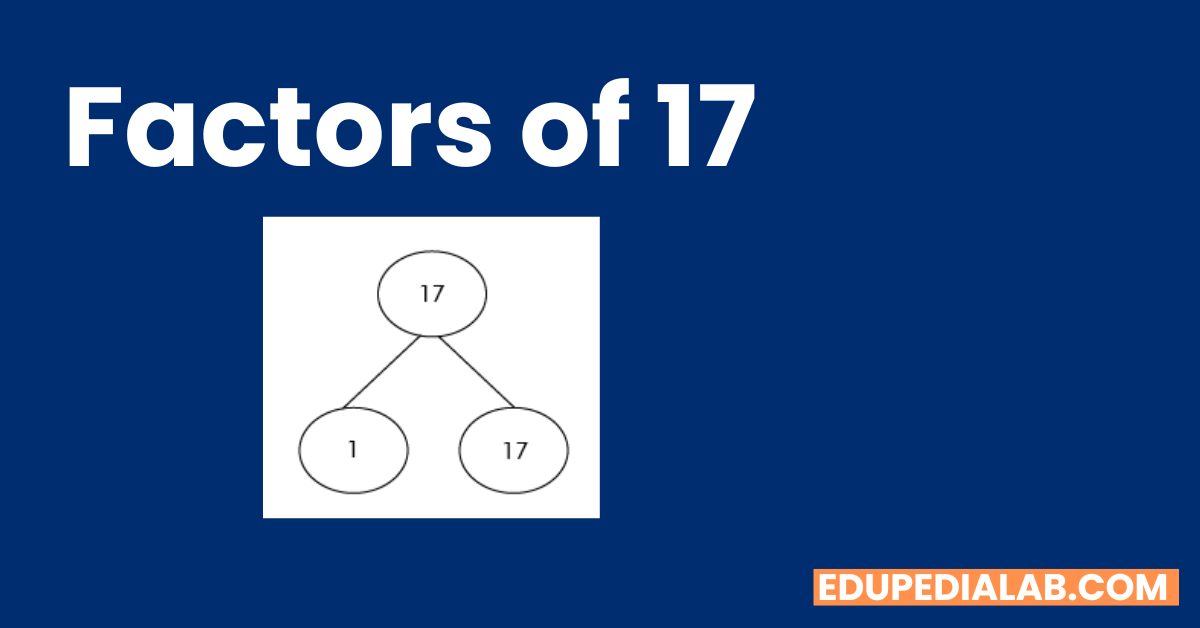
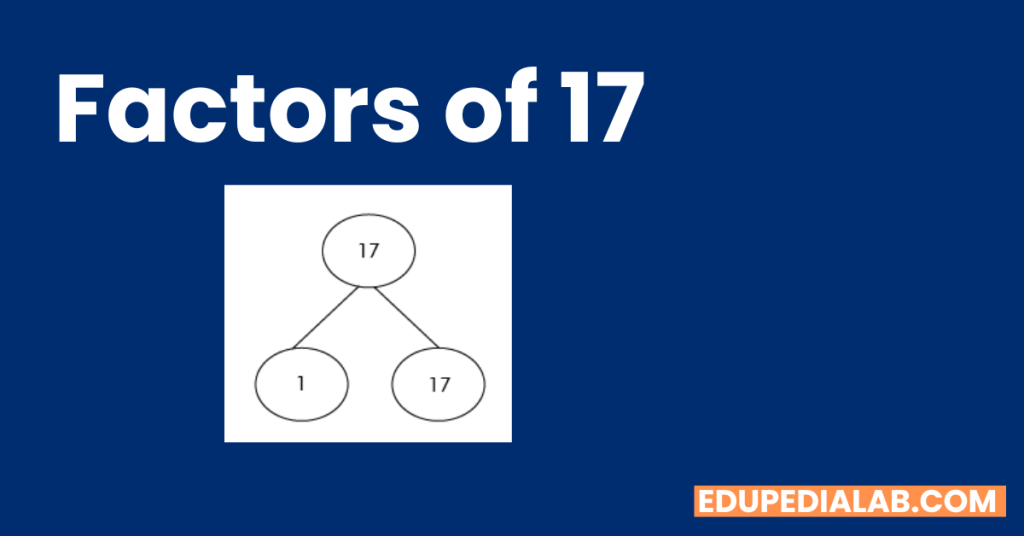
The factors of 17 are 1 and 17. Since 17 is a prime number, it has no other factors. It cannot be divided evenly by any other number.
5. The Significance of 17 as a Prime Number
As a prime number, 17 holds a special place in mathematics. Prime numbers are the building blocks of all natural numbers, and they have unique properties that make them essential in various fields, including cryptography, number theory, and algorithms. The fact that 17 is a prime number contributes to its significance and mathematical intrigue.
6. Properties of 17
Apart from being a prime number, 17 possesses certain properties that make it interesting to mathematicians. Some of its notable properties include:
- It is an odd number.
- It is a positive integer.
- It is a single-digit prime number.
- It is not a perfect square.
7. Applications of 17 in Mathematics
The presence of prime numbers like 17 is crucial in many mathematical concepts and applications. Some areas where 17 finds relevance include:
- Cryptography and encryption algorithms.
- Prime factor
- Prime factorization and prime factor analysis.
- Sieve of Eratosthenes for finding prime numbers.
- Number theory and prime number patterns.
- The unique properties of 17 contribute to its significance in these mathematical applications, making it an important number to study and understand.
17 and Number Theory
Number theory, a branch of mathematics that deals with the properties and relationships of numbers, finds 17 to be an intriguing subject. Mathematicians explore the patterns, divisibility rules, and prime factorization of 17 to uncover deeper insights into number theory concepts.
9. 17 in Everyday Life
While the number 17 may not have as much practical significance in everyday life as other numbers, it does appear in various contexts. For instance, in sports, the number 17 is often assigned to athletes or used to represent a particular position on a team. Additionally, it may hold personal significance for individuals in different cultures and traditions.
10. Prime Factorization of 17
As mentioned earlier, 17 is a prime number, which means it cannot be factored into smaller integers. Its prime factorization is simply 17. This characteristic makes it distinct from composite numbers, which can be expressed as products of prime factors.
11. Factors vs. Multiples
While factors refer to the numbers that divide another number evenly, multiples are the numbers obtained by multiplying a given number by an integer. For example, the multiples of 17 are 17, 34, 51, 68, and so on. The factors of 17 are different from its multiples, as they focus on division rather than multiplication.
12. Interesting Patterns in the Factors of 17
The factors of 17 may seem simple at first glance, with only two factors: 1 and 17. However, mathematicians often uncover intriguing patterns and relationships within the factors of prime numbers. Exploring these patterns can lead to new discoveries and further understanding of number theory.
13. Factors of 17 in Different Number Bases
Number bases, such as decimal (base-10), binary (base-2), and hexadecimal (base-16), represent numbers using different symbols and positional values. The factors of 17 remain the same regardless of the number base used. In other words, 17 will always have factors of 1 and 17, regardless of the number system.
14. Relations between Factors of 17 and Other Prime Numbers
Studying the relationships between the factors of 17 and other prime numbers can provide insights into prime number distribution and patterns. Mathematicians analyze the interplay between different primes to unravel connections and deepen their understanding of number theory.
Conclusion
In conclusion, the factors of 17, being a prime number, consist of only 1 and 17. Despite its simplicity, 17 holds significance in mathematics, contributing to various areas such as cryptography, number theory, and prime factorization. Exploring the properties and relationships of 17 allows mathematicians to uncover new insights and expand their knowledge of the intricate world of numbers.
FAQs
- Q: Are the factors of 17 limited to 1 and 17?
- A: Yes, as a prime number, 17 only has two factors: 1 and 17.
- Q: Can the factors of 17 be expressed as a product of other numbers?
- A: No, the factors of 17 cannot be further divided into smaller integers.
- Q: What are some practical applications of studying the factors of 17?
- A: Studying the number 17 has crucial application in number theory.
- Q: How does 17 contribute to cryptography?
- A: Prime numbers like 17 are essential in cryptography algorithms for encryption and data security. They help ensure the strength and complexity of encryption methods.
- Q: Can the factors of 17 be used in number puzzles or games?
- A: Yes, the factors of 17 can be incorporated into number puzzles or games that involve divisibility or prime numbers. They can provide interesting challenges and opportunities for problem-solving.
- Q: Are there any known theorems or conjectures specifically related to the factors of 17?
- A: Currently, there are no widely known theorems or conjectures exclusively focused on the factors of 17. However, ongoing research in number theory may bring new discoveries in the future.
- Q: Is 17 a significant number in any cultural or historical contexts?
- A: While 17 may not hold specific cultural or historical significance like some other numbers, it may have personal or symbolic importance to individuals in different contexts.
- Q: Can the factors of 17 be calculated using mathematical formulas or algorithms?
- A: Since the factors of 17 are limited to 1 and 17, they can be easily identified through simple division. No complex formulas or algorithms are required.
Conclusion
In conclusion, the factors of 17, being a prime number, consist of only 1 and 17. Despite its simplicity, 17 holds significance in mathematics, contributing to various areas such as cryptography, number theory, and prime factorization. Exploring the properties and relationships of 17 allows mathematicians to uncover new insights and expand their knowledge of the intricate world of numbers.
We have seen that 17 is a prime number with unique characteristics, making it a fascinating subject of study. Its role in cryptography, number theory, and other mathematical applications demonstrates its relevance and importance in various fields. By understanding the factors of 17 and their implications, we gain a deeper appreciation for the rich tapestry of mathematics.