Factors of 100 (Explained By Experts)
Factors of 100
What is Factorization?
Factorization is the process of breaking down a mathematical object, typically a number or polynomial, into its constituent factors. In the case of numbers, factorization involves finding the prime factors of a number, while in the case of polynomials, it involves expressing the polynomial as a product of simpler polynomials or irreducible factors. The Factors of 100 are 1, 2, 4, 5, 10, 20, 25, 50, and 100.
For example, the number 12 can be factored as 2 x 2 x 3, where 2 and 3 are prime factors. Similarly, the polynomial x^2 + 2x + 1 can be factored as (x + 1)^2, which is a product of two identical factors. Factorization is an important concept in many areas of mathematics, including algebra, number theory, and cryptography.
What are the Factors in Mathematics?
In mathematics, a factor is a number or polynomial that divides another number or polynomial evenly, without leaving a remainder. More formally, if a and b are two numbers or polynomials, then b is a factor of an if there exists another number or polynomial c such that a = b × c.
For example, the number 12 can be factored as 2 × 2 × 3, where 2 and 3 are factors of 12. Similarly, the polynomial x^2 + 2x + 1 can be factored as (x + 1) × (x + 1), where x + 1 is a factor of the polynomial.
Factors play an important role in many areas of mathematics, including algebra, number theory, and geometry. In particular, finding the factors of a number or polynomial can help us to understand its properties and relationships to other mathematical objects. You can use different means including the division method, factor tree, or prime factorization to find out factors of 200.
How to find out the factorization of any decimal number?
To find the factorization of a decimal number, you first need to convert it into a whole number by multiplying both the numerator and denominator of the decimal by a power of 10. For example, to convert the decimal 0.75 into a whole number, you can multiply it by 100 to get 75.
Once you have converted the decimal into a whole number, you can then find its factorization using the following steps:
- Find the prime factors of the whole number. This can be done by dividing the number by the smallest prime number (2, 3, 5, 7, etc.) that divides it evenly, and then dividing the resulting quotient by the smallest prime factor that divides it evenly, and so on until you reach 1.
- Write the prime factors as a product of their powers. For example, if the prime factors of the number are 2, 3, and 5, and each factor occurs twice, then the factorization of the number can be written as 2^2 x 3^2 x 5^2.
- If the original decimal number had a denominator that was not a power of 10, you can simplify the factorization by dividing each factor by the appropriate power of 10. For example, if the original decimal was 0.75, then its factorization as a whole number would be 75 = 3 x 5^2, and its factorization as a decimal would be 3/10 x 5/10^2 = 3/10 x 1/20 = 3/200.
Note that not all decimal numbers have a finite factorization, as some decimals are irrational and cannot be expressed as a finite product of prime factors.
Types of Factors
There are several types of factors in mathematics:
- Prime factor: A prime factor is a factor that is also a prime number. Prime factors can only be divided evenly by 1 and themselves. For example, the prime factors of 12 are 2 and 3, because 2 and 3 are both prime numbers, and 2 × 2 × 3 = 12.
- Composite factor: A composite factor is a factor that is not a prime number. Composite factors can be divided evenly by more than just 1 and themselves. For example, 4 is a composite factor of 12, because it is not a prime number, and 4 × 3 = 12.
- Co-factor: A cofactor of a number or polynomial is a factor that is not the number or polynomial itself. For example, the co-factors of 12 are all the factors of 12 except 12 itself.
- Proper factor: A proper factor of a number or polynomial is a factor that is less than the number or polynomial itself. For example, the proper factors of 12 are 1, 2, 3, 4, and 6, but not 12.
- Common factor: A common factor of two or more numbers or polynomials is a factor that divides all of them evenly. For example, 3 is a common factor of 6 and 9, because both 6 and 9 can be divided evenly by 3.
- Greatest common factor: The greatest common factor (GCF) of two or more numbers or polynomials is the largest factor that divides all of them evenly. For example, the GCF of 12 and 18 is 6, because 6 is the largest factor that divides both 12 and 18 evenly.
Factors of 100
To find out the factors of 100, we can start by listing out the positive integers that divide evenly into 100. These are known as the factors of 100.
- One way to find the factors is to list out all the positive integers less than or equal to the square root of 100, which is 10:1, 2, 4, 5, 10Note that we only need to list the factors up to the square root of 100, because any factors larger than the square root will have a corresponding factor that is smaller than the square root. For example, if 20 is a factor of 100, then 5 is also a factor because 20 x 5 = 100.
- We can also find the factors by dividing 100 by each of its positive integer divisors. For example:100 ÷ 1 = 100, 100 ÷ 2 = 50 100 ÷ 4 = 25 100 ÷ 5 = 20 100 ÷ 10 = 10So the factors of 100 are 1, 2, 4, 5, 10, 20, 25, 50, and 100.
Note that 1 and 100 are always factors of any number, and any other factors come in pairs (except for the square root if the number is a perfect square).
| Number | Factor? | Calculation |
|---|---|---|
| 1 | Yes | 100 ÷ 1 = 100 |
| 2 | Yes | 100 ÷ 2 = 50 |
| 3 | No | |
| 4 | Yes | 100 ÷ 4 = 25 |
| 5 | Yes | 100 ÷ 5 = 20 |
| 6 | No | |
| 7 | No | |
| 8 | No | |
| 9 | No | |
| 10 | Yes | 100 ÷ 10 = 10 |
| 11 | No | |
| 12 | No | |
| 13 | No | |
| 14 | No | |
| 15 | No | |
| 16 | No | |
| 17 | No | |
| 18 | No | |
| 19 | No | |
| 20 | Yes | 100 ÷ 20 = 5 |
| 25 | Yes | 100 ÷ 25 = 4 |
| 50 | Yes | 100 ÷ 50 = 2 |
| 100 | Yes | 100 ÷ 100 = 1 |
In this table, we list the numbers from 1 to 100 and check whether they are factors of 100. To determine if a number is a factor of 100, we divide 100 by the number and check if the result is a whole number (without any remainder). If the result is a whole number, then the number is a factor of 100. We list the factors in the “Factor?” column and show the calculation used to find each factor in the “Calculation” column. In this case, we find that the factors of 100 are 1, 2, 4, 5, 10, 20, 25, 50, and 100.

Summary
Factorization of 100 involves finding the set of integers that multiply together to equal 100. The factors of 100 are 1, 2, 4, 5, 10, 20, 25, 50, and 100. 100 can be written as a product of prime factors, which are 2 × 2 × 5 × 5, or 2^2 × 5^2. Factorization is a useful tool in number theory and can help us to solve various mathematical problems.

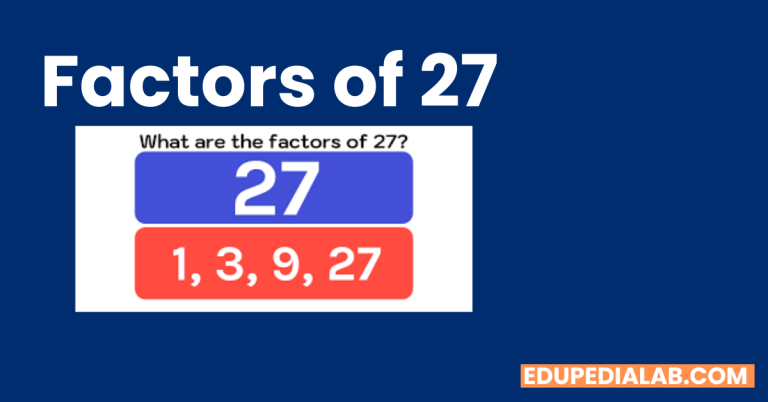

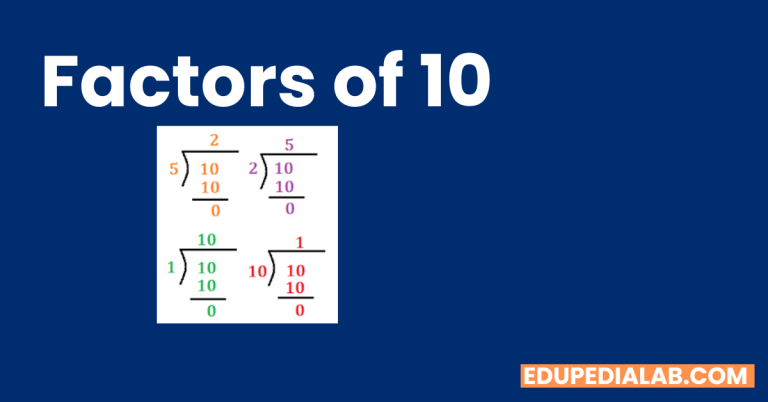
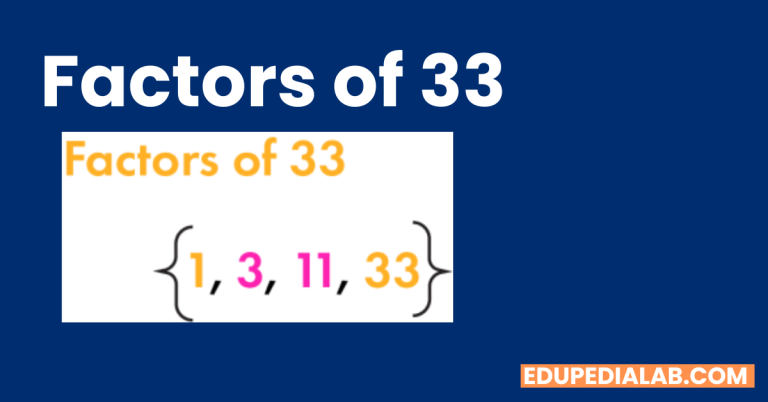
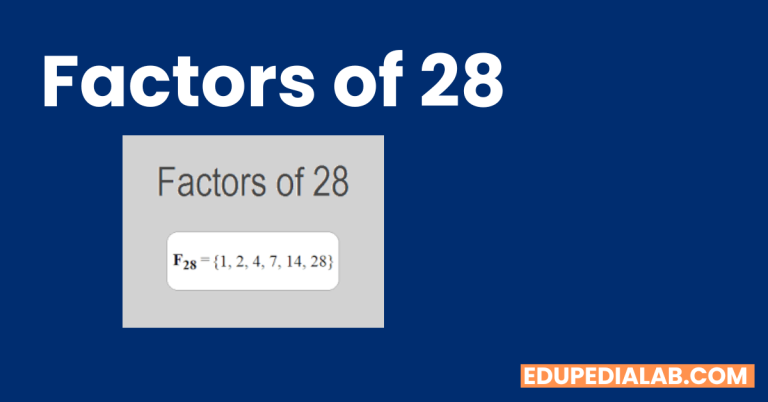
3 Comments