Factors of 12 (Explained By Experts)
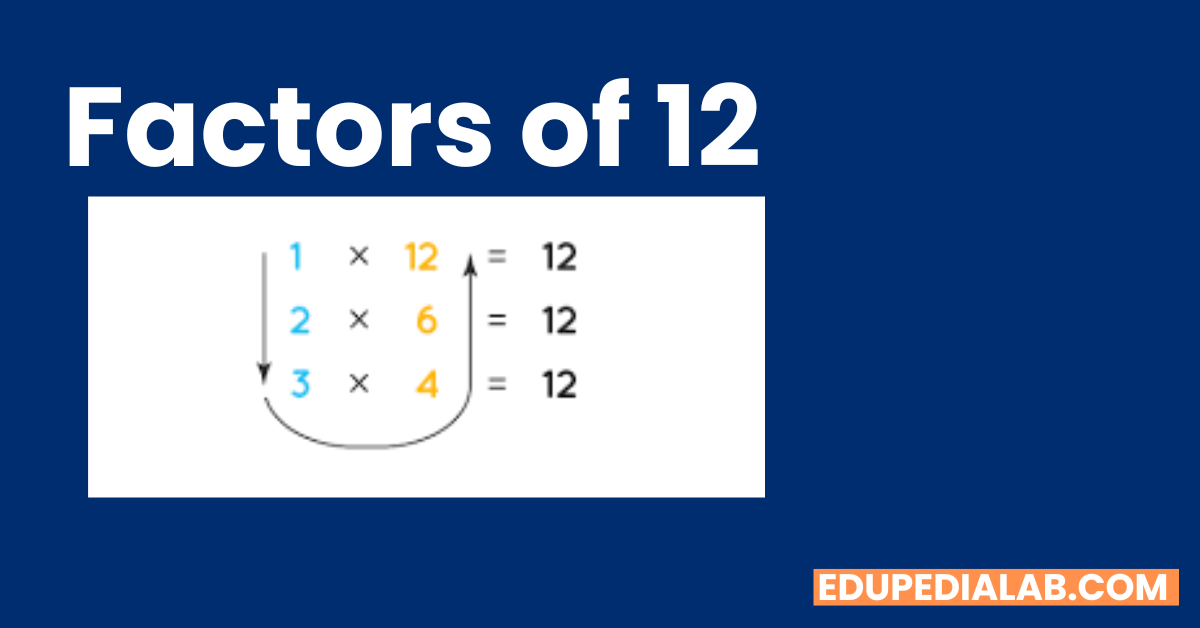
Factors of 12
In mathematics, factors play a crucial role in understanding the properties and relationships of numbers. Factors are the numbers that can be multiplied together to obtain a given number. In this article, we will explore the factors of 12, a small yet significant number in the world of mathematics.
Factors are numbers that divide a given number without leaving a remainder. They provide valuable insights into the mathematical properties and relationships of numbers. In this article, we will delve into the factors of 12, a number that has its own unique set of factors.
What are the Factors?
Factors are the numbers that can be multiplied together to obtain a particular number. For example, the factors of 12 are the numbers that, when multiplied, give the product 12. These factors can be positive or negative integers, but for simplicity, we will focus on positive factors in this article.
Finding Factors of 12
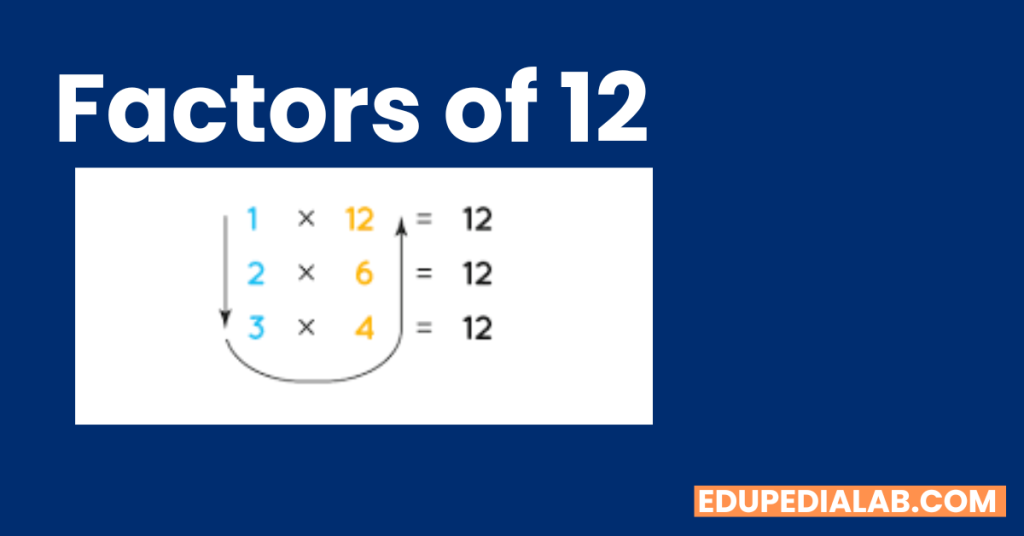
To find the factors of 12, we can systematically divide 12 by different numbers and check if they evenly divide it. Let’s explore the divisibility of 12 by various numbers:
Divisibility by 1 and 12
Every number is divisible by 1 and itself. Therefore, 1 and 12 are always factors of 12.
Divisibility by 2
To check if 12 is divisible by 2, we examine whether it leaves a remainder when divided by 2. Since 12 is an even number, it is divisible by 2. Therefore, 2 is a factor of 12.
Divisibility by 3
We can determine the divisibility of 12 by 3 by calculating the sum of its digits. In this case, 1 + 2 = 3, which is divisible by 3. Hence, 3 is a factor of 12.
Divisibility by 4
For a number to be divisible by 4, the last two digits of the number must be divisible by 4. In the case of 12, the last two digits are 12, which is divisible by 4. Consequently, 4 is a factor of 12.
Divisibility by 6
Since 12 is divisible by both 2 and 3, it is also divisible by 6. Hence, 6 is a factor of 12.
Prime Factorization of 12
Prime factorization is the process of expressing a number as the product of its prime factors. To determine the prime factorization of 12, we can factorize it as 2 × 2 × 3 or 2² × 3.
Applications of Factors of 12
The factors of 12 find applications in various mathematical concepts. Some of the notable applications include:
- Algebraic Manipulations: Factors of 12 play a vital role in simplifying algebraic expressions and solving equations.
- Number Systems: Factors help in understanding the properties of different number systems, such as integers and rational numbers.
- Multiples and Divisibility: The factors of 12 aid in determining the multiples of 12 and the divisibility rules associated with it. For example, if a number is divisible by both 3 and 4, it is also divisible by 12.
- Fractions and Ratios: Factors of 12 help in simplifying fractions and calculating ratios. By dividing the numerator and denominator of a fraction by their common factors with 12, we can obtain an equivalent fraction in its simplest form.
- Geometry: Factors of 12 can be useful in geometry when dealing with measurements, angles, and ratios in different shapes and figures.
- Understanding the factors of 12 provides a foundation for various mathematical concepts and applications. By recognizing these factors, we can unlock the relationships and patterns that exist within numbers.
You can easily check the Factors Of 24 by using the division or prime factorization process.
Conclusion
In conclusion, the factors of 12 are the numbers that can divide 12 without leaving a remainder. These factors include 1, 2, 3, 4, 6, and 12. The prime factorization of 12 is expressed as 2² × 3. The factors of 12 have practical applications in algebra, number systems, multiples, fractions, ratios, and geometry. By comprehending the factors of 12, we gain valuable insights into the properties and relationships of numbers in mathematics.