Factors Of 15 (Explained By Experts)
Factors OF 15
In mathematics, factors and multiples play a significant role in understanding the properties of numbers. In this article, we will delve into the factors of 15, examining the divisors and multiples that make up this number. Understanding the factors of 15 will not only enhance your knowledge of mathematics but also provide you with a solid foundation for solving more complex problems. So, let’s dive in and explore the fascinating world of factors of 15. When you calculate the Factors Of 30 then you will see the number 15 among them.
Factors Of 15
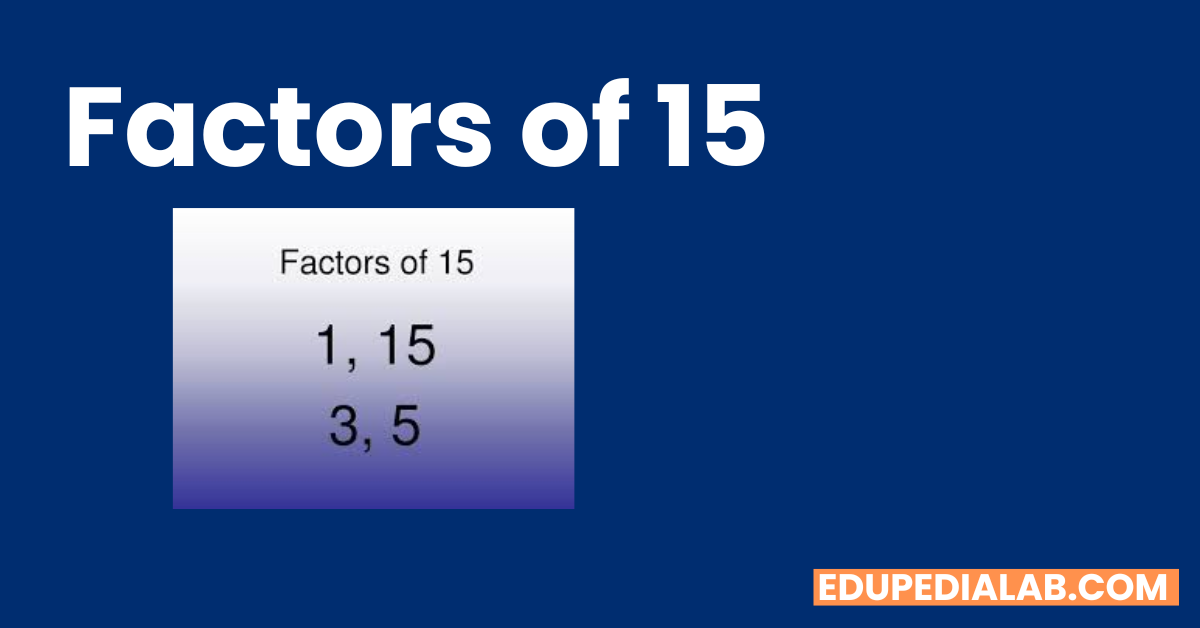
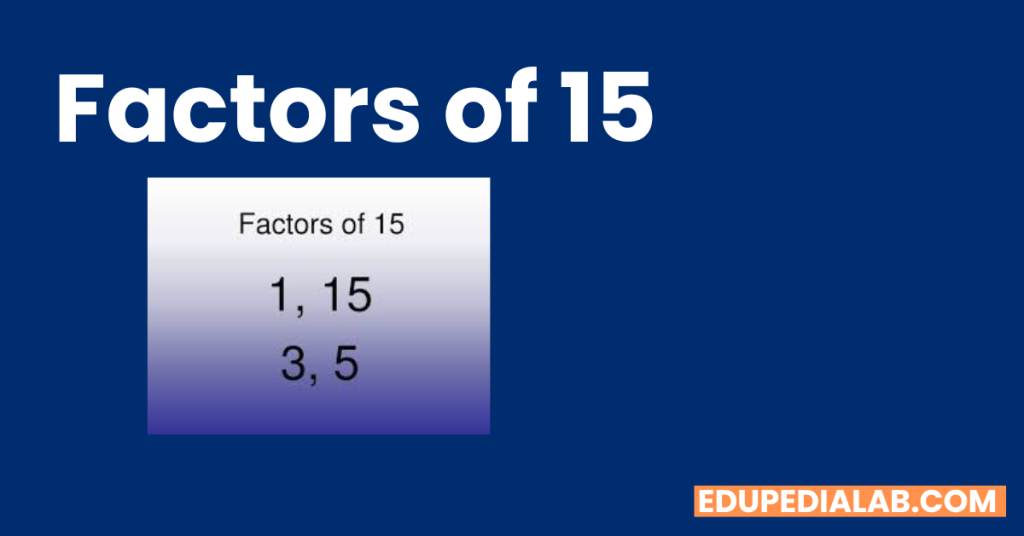
The factors of 15 are the numbers that divide evenly into 15 without leaving a remainder. In other words, they are the divisors of 15. By finding the factors of 15, we can gain insights into the number’s properties and relationships with other numbers. Let’s list the factors of 15 below:
- 1: 15 ÷ 1 = 15
- 3: 15 ÷ 3 = 5
- 5: 15 ÷ 5 = 3
- 15: 15 ÷ 15 = 1
As we can see, the factors of 15 are 1, 3, 5, and 15. These numbers evenly divide 15, resulting in whole numbers. Now that we have identified the factors, let’s explore some interesting properties and applications of the factors of 15.
Properties of Factors Of 15
Prime Factorization of 15
To find the prime factorization of 15, we express it as a product of prime numbers. The prime factorization of 15 is given by:
15 = 3 × 5
Here, both 3 and 5 are prime numbers. Prime factorization is useful in various mathematical applications, such as simplifying fractions or finding the least common multiple (LCM) and greatest common divisor (GCD) of numbers.
Factors and Multiples Relationship
The factors and multiples of a number are closely related. The factors of 15 divide 15 evenly, while the multiples of 15 are obtained by multiplying 15 with other numbers. Let’s explore the relationship between factors and multiples of 15:
Factors
- Factors of 15: 1, 3, 5, 15
Multiples
- Multiples of 15: 15, 30, 45, 60, …
As we can see, the multiples of 15 are obtained by multiplying 15 with any whole number, resulting in numbers that are divisible by 15. Understanding the relationship between factors and multiples helps us solve problems involving divisibility and arithmetic operations.
Applications of Factors Of 15
Finding Common Factors
The factors of 15 can be used to find the common factors of multiple numbers. By identifying the common factors, we can determine the highest common factor (HCF) or greatest common divisor (GCD) shared by those numbers. Let’s consider an example:
Example: Find the common factors of 15, 20, and 25.
Solution:
The factors of 15 are 1, 3, 5, and 15. The factors of 20 are 1, 2, 4, 5, 10, and 20. The factors of 25 are 1, 5, and 25.
From the lists above, we can see that the common factors of 15, 20, and 25 are 1 and
- Therefore, the highest common factor (HCF) or greatest common divisor (GCD) of 15, 20, and 25 is 5.
In this example, the factors of 15 help us identify the common factors shared with other numbers, enabling us to determine their highest common factor. This concept is crucial in various mathematical applications, including simplifying fractions and solving equations.
Divisibility Rules
Understanding the factors of 15 allows us to apply divisibility rules. Divisibility rules help us determine if a number is divisible by another without performing the actual division. Let’s explore some divisibility rules related to the factors of 15:
- Divisibility by 1: Every number is divisible by 1.
- Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3. For example, let’s consider the number 153. The sum of its digits is 1 + 5 + 3 = 9, which is divisible by 3. Therefore, 153 is divisible by 3.
- Divisibility by 5: A number is divisible by 5 if its units digit is either 0 or 5. For example, 15 ends with the digit 5, making it divisible by 5.
By utilizing these divisibility rules, we can quickly determine if a given number is divisible by 15 or any of its factors.
FAQs (Frequently Asked Questions)
Q1: What are the prime factors of 15?
A1: The prime factors of 15 are 3 and 5.
Q2: Is 15 a composite number?
A2: Yes, 15 is a composite number because it has factors other than 1 and itself.
Q3: How can I find the factors of any number?
A3: To find the factors of any number, start by dividing it by the smallest prime numbers (2, 3, 5, 7, …) and continue dividing until you reach the number itself. The divisors obtained during this process are the factors of the given number.
Q4: What is the significance of finding factors of a number?
A4: Finding factors helps us understand the divisibility and relationships between numbers. It is particularly useful in simplifying fractions, solving equations, and identifying common factors.
Q5: Can the factors of 15 be negative numbers?
A5: No, the factors of 15 are always positive integers because negative numbers do not divide evenly into 15.
Q6: How can I use the factors of 15 to find other multiples?
A6: By multiplying the factors of 15 with different numbers, you can find multiples of 15. For example, multiplying 15 by 2 gives you a multiple of 30 (15 × 2 = 30).
Conclusion
Understanding the factors of 15 is essential for gaining insights into the properties and relationships of numbers. By exploring the divisors and multiples of 15, we have uncovered the prime factors, divisibility rules, and applications of these factors. The factors of 15 not only contribute to the foundations of mathematics but also play a role in various real-life applications. So, the next time you encounter the number 15, remember its factors and the wealth of knowledge they provide.