Factors of 27 (Explained By Experts)
Factors of 27
Welcome to this comprehensive guide on the factors of 27. In this article, we will delve into the fascinating world of numbers and explore the different factors that make up the number 27. Whether you’re a math enthusiast, a student, or simply curious about the wonders of mathematics, this article will provide you with a deep understanding of the factors of 27. So, let’s embark on this numerical journey together. The common thing about Factors OF 27 and Factors Of 18 are 1,3 and number 9.
Factors Of 27 Explained
To begin our exploration, let’s first define what factors are. Factors are the whole numbers that can be multiplied together to produce a given number. In the case of 27, the factors are the numbers that can be multiplied together to yield the result of 27. In other words, they are the building blocks of this particular number.
Prime Factorization of 27
To determine the factors of 27, it is essential to understand the concept of prime factorization. Prime factorization involves breaking down a number into its prime factors, which are the prime numbers that can divide the given number without leaving a remainder.
In the case of 27, its prime factorization can be expressed as 3 × 3 × 3 or 3^3. This means that 3 is the only prime factor of 27, and it appears three times in the factorization.
List of Factors
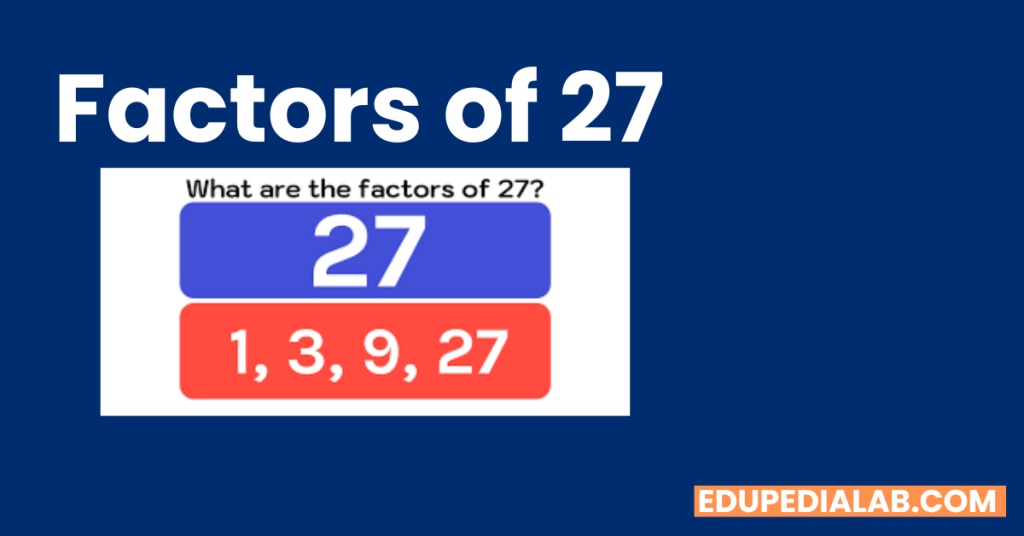
Now that we understand the prime factorization of 27, let’s list out all the factors of 27:
- 1
- 3
- 9
- 27
As you can see, the factors of 27 are 1, 3, 9, and 27. These numbers can be multiplied together in various combinations to result in 27. It’s interesting to note that 27 is divisible by both 1 and itself, which is a characteristic of all numbers.
Properties of Factors
Factors possess several interesting properties that make them valuable in various mathematical operations. Here are some notable properties of factors:
- Multiplication: When you multiply two factors of a number, the result is always the original number. For example, 3 × 9 = 27.
- Divisibility: If a number is divisible by another number, the divisor is always a factor of the original number. For instance, 27 is divisible by 3, which means 3 is a factor of 27.
- Factor Pairs: Factors can be paired to form the original number. In the case of 27, the factor pairs are (1, 27) and (3, 9).
- Commutativity: The order in which factors are multiplied does not affect the result. For example, 3 × 9 is the same as 9 × 3, and both equal 27.
Applications of Factors
Factors find applications in various areas of mathematics and beyond. Here are a few examples:
- Number Theory: Factors play a fundamental role in number theory, the branch of mathematics that deals with the properties and relationships of numbers. Understanding factors helps in analyzing the properties of numbers and solving mathematical problems.
- Prime Numbers: Factors are closely related to prime numbers, which have only two factors: 1 and the number itself. Prime numbers are crucial in cryptography, number theory, and many computational algorithms.
- Common Factors: Identifying common factors between two or more numbers is useful in simplifying fractions, finding the greatest common divisors, and determining common multiples.
- Algebraic Expressions: Factors are essential when factoring algebraic expressions. By factoring, we can simplify complex expressions, solve equations, and analyze the behavior of polynomials.
- Problem-solving: Factors provide a foundation for problem-solving in mathematics. By understanding the factors of a number, we can break down complex problems into simpler components and devise efficient strategies to find solutions.
Frequently Asked Questions (FAQs)
- Q: Can 27 be evenly divided by any other number apart from 1 and itself?
- Yes, 27 can be evenly divided by 3, resulting in a quotient of 9.
- Q: Are there any other numbers with the same set of factors as 27?
- No, 27 is a unique number with its own distinct set of factors.
- Q: How can I find the factors of a larger number?
- To find the factors of a larger number, start by dividing it by the smallest prime numbers (2, 3, 5, 7, etc.) until you cannot divide any further. The resulting quotients and the original number itself are the factors.
- Q: What is the significance of prime factors in factorization?
- Prime factors are crucial in determining the unique set of factors for a number. By identifying the prime factors and their exponents, we can express the number as a product of primes, providing valuable insights into its properties.
- Q: How can knowledge of factors be applied in real-life scenarios?
- Understanding factors helps in various practical situations. For instance, in manufacturing, factors are used to determine the optimal arrangement of items on a production line. In finance, factors are employed in calculating interest rates, investments, and loan repayments.
- Q: Are factors only applicable to whole numbers?
- Factors are primarily associated with whole numbers, but the concept can be extended to include fractions and even irrational numbers in certain contexts.
Conclusion
In conclusion, the factors of 27 are the numbers that, when multiplied together, yield the result of 27. By understanding the prime factorization of 27, which consists of the prime number 3 raised to the power of 3, we can identify the factors as 1, 3, 9, and 27. Factors play a significant role in mathematics, serving as building blocks for more complex calculations and providing insights into the properties of numbers.
Whether you’re exploring number theory, solving equations, or analyzing real-world scenarios, a solid understanding of factors is invaluable. So, embrace the world of factors, unlock the mysteries of multiples, and embark on a numerical adventure that will deepen your mathematical knowledge.