Factors of 28 (Explained By Experts)

Factors of 28

When it comes to understanding the properties of numbers, exploring their factors is an essential concept. Factors are the numbers that can be multiplied together to produce a given number. In this article, we will delve into the factors of 28, breaking down this number and identifying its divisors. By the end, you will have a comprehensive understanding of the factors that contribute to the number 28. The numbers 1, 2, 7, and 14 are the Factors Of 14.

1. Understanding Factors and Divisors

Before diving into the specific factors of 28, let’s first establish a clear understanding of factors and divisors. Factors are the numbers that divide evenly into a given number without leaving a remainder. Divisors, on the other hand, are the numbers by which a given number is divisible. In simpler terms, factors are the building blocks of a number, whereas divisors are the numbers that divide the given number evenly.

2. Prime Factorization of 28

To find the prime factorization of 28, we need to determine the prime numbers that, when multiplied together, give us the number 28. Prime numbers are numbers greater than 1 that have no divisors other than 1 and themselves. In the case of 28, its prime factorization is 2 * 2 * 7.

3. Divisors of 28

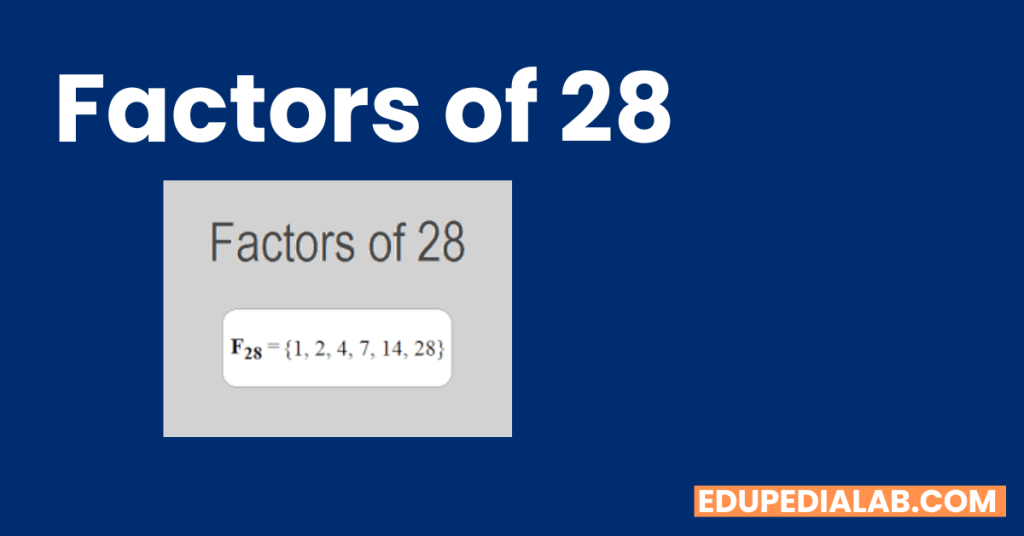
Now that we have determined the prime factorization of 28, let’s identify its divisors. The divisors of 28 are the numbers that divide 28 without leaving a remainder. In the case of 28, the divisors are 1, 2, 4, 7, 14, and 28.

4. Properties of the Factors of 28

The factors of 28 possess several interesting properties. Firstly, the sum of the factors of 28, excluding the number itself, is equal to the number itself (1 + 2 + 4 + 7 + 14 = 28). This property holds true for perfect numbers. Additionally, 28 is an abundant number since the sum of its proper divisors (1 + 2 + 4 + 7 + 14 = 28) is greater than the number itself.

5. Applications of Factors in Mathematics

Factors play a crucial role in various mathematical applications. They are used in prime factorization, simplifying fractions, solving equations, finding common factors, and determining divisors or multiples of numbers. Factors also aid in understanding the relationships between numbers and help in solving real-world problems.

6. Real-Life Examples of Factors

The concept of factors extends beyond the realm of mathematics and finds applications in our daily lives. For instance, when calculating the number of seats in a theater, factors help determine the various seating arrangements that can accommodate different audience sizes. Factors are also utilized in engineering and architecture to design structures that can withstand different loads and stresses. Additionally, factors are relevant in financial planning, where they assist in calculating interest rates, loan repayments, and investment returns.

7. The Importance of Prime Factors

Prime factors hold significant importance in mathematics. They help us understand the fundamental building blocks of numbers and their unique characteristics. Prime factorization allows us to express a number as a product of prime numbers, providing valuable insights into the number’s properties and relationships.

8. Divisibility Tests for 28

Divisibility tests are useful tools to determine if a number is divisible by another number without performing division. In the case of 28, we can use certain rules to check for divisibility. For example, 28 is divisible by 2 since it is an even number. Furthermore, if the sum of the digits in a number is divisible by 3, the number itself is divisible by 3. Applying this rule to 28 (2 + 8 = 10), we can conclude that 28 is not divisible by 3.

9. The Relationship Between Factors and Multiples

Factors and multiples are closely related concepts. Factors are the numbers that divide evenly into a given number, while multiples are obtained by multiplying a number by another integer. For example, the multiples of 28 are 28, 56, 84, 112, and so on, while the factors of 28 are 1, 2, 4, 7, 14, and 28.

10. Factors of 28 in Other Number Systems

The factors of 28 remain the same regardless of the number system used. Whether in decimal, binary, octal, or hexadecimal systems, the divisors of 28 will always be 1, 2, 4, 7, 14, and 28. However, the representation of these numbers may differ in each system.

11. Interesting Facts about the Factors of 28

Here are some fascinating facts about the factors of 28:

• 28 is a perfect number, as the sum of its factors (excluding itself) is equal to the number itself.
• The factors of 28 can be grouped into pairs: (1, 28) and (2, 14), where the product of each pair is 28.
• 28 is a composite number since it has factors other than 1 and itself.
• The prime factors of 28 are 2 and 7.

12. Mathematical Operations with Factors

Factors play a vital role in various mathematical operations. For example, when multiplying two numbers, the product will have factors that include the factors of both numbers. Similarly, when dividing a number by another number, the factors of the divisor are also factors of the quotient.

13. The Role of Factors in Algebra

In algebra, factors are crucial in factoring polynomials. By factoring, we can break down a polynomial into its constituent factors, making it easier to analyze and solve equations. The process of finding common factors in algebraic expressions is also based on the concept of factors.

14. Factors of 28 and Their Significance in Number Theory

In number theory, the study of factors plays a significant role. The factors of 28 are analyzed to understand their properties, relationships, and patterns. Number theorists examine the distribution of factors, their divisibility properties, and their implications in various mathematical contexts.

15. Conclusion

In conclusion, the factors of 28 are the numbers that divide evenly into 28 without leaving a remainder. By exploring the divisors and properties of these factors, we gain a deeper understanding of the number 28 and its mathematical significance.