Factors of 33 (Explained By Experts)
Factors of 33
Factors are the numbers that can be multiplied together to give a particular number. In the case of 33, it is important to understand its factors, which are the numbers that divide evenly into 33 without leaving a remainder. By exploring the factors of 33, we can gain insights into its mathematical properties and relationships. In this article, we will delve into the factors of 33 and examine their significance. If you have mathematical curiosity you might be looking for ways to know the Factors Of 11. The number 11 is very much unique in many senses and is one of the factors of 33.
Understanding Factors
Before we dive into the factors of 33, let’s have a brief understanding of factors in general. Factors are whole numbers that can be multiplied together to obtain a given number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12, as these numbers can be multiplied in various combinations to yield 12 (1 x 12, 2 x 6, 3 x 4). Factors play a crucial role in many mathematical concepts and calculations.
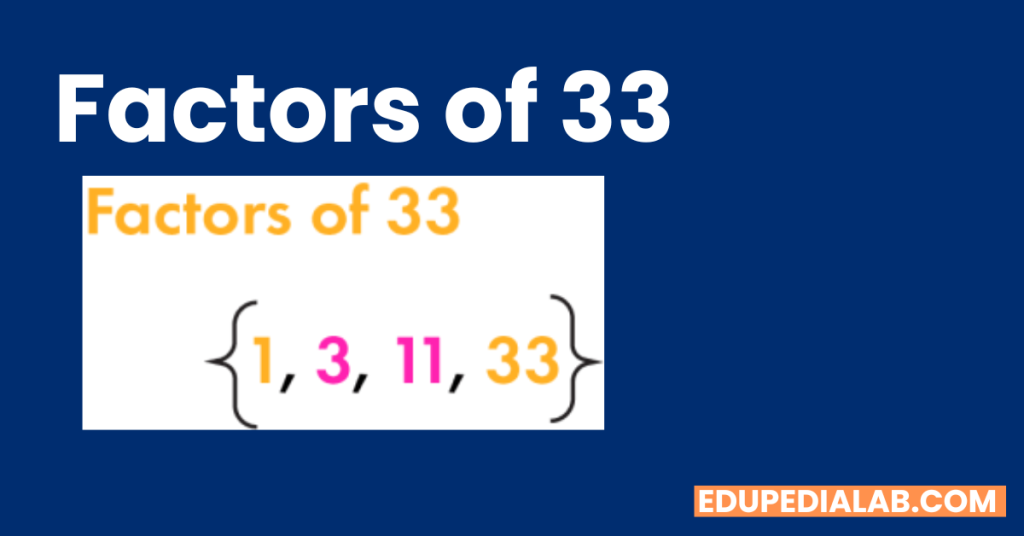
The Factors of 33
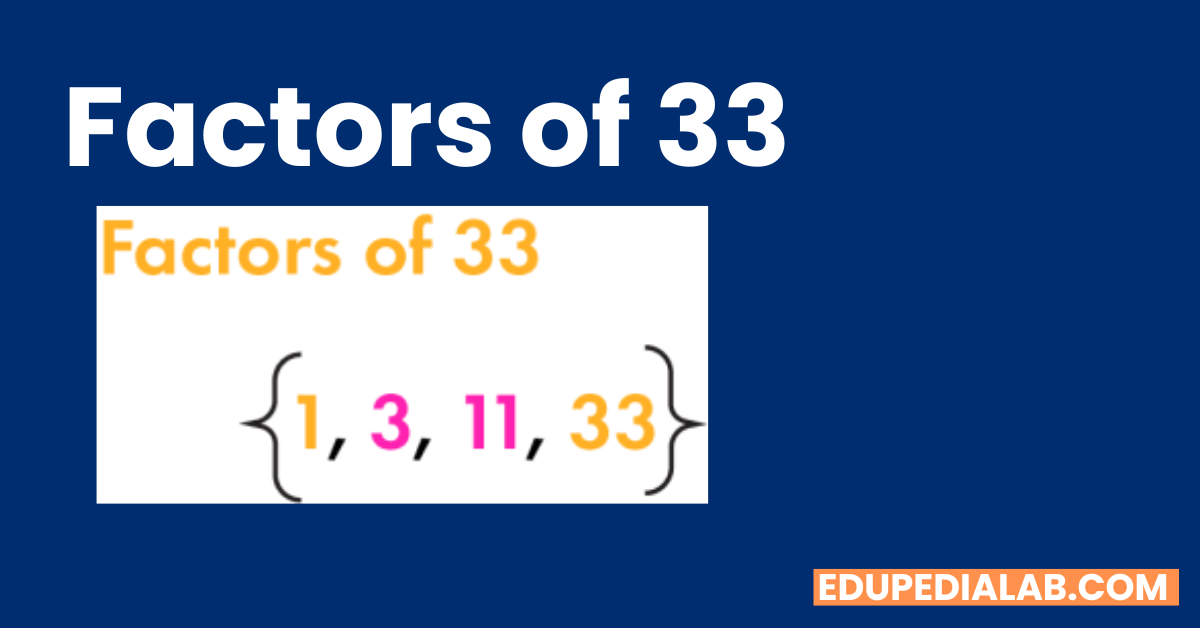
To find the factors of 33, we need to identify the numbers that divide 33 evenly. Let’s examine the factors of 33:
- Factor 1: 33 ÷ 1 = 33
- Factor 3: 33 ÷ 3 = 11
- Factor 11: 33 ÷ 11 = 3
- Factor 33: 33 ÷ 33 = 1
Therefore, the factors of 33 are 1, 3, 11, and 33. These are the numbers that can be multiplied together to yield 33.
Properties of 33 and Its Factors
Now that we know the factors of 33, let’s explore some interesting properties and relationships associated with them.
Prime Factorization
Prime factorization is the process of expressing a number as a product of prime numbers. To determine the prime factorization of 33, we need to express it as a product of its prime factors. In the case of 33, the prime factors are 3 and 11. Therefore, the prime factorization of 33 is 3 x 11.
Divisibility Rules
The factors of 33 provide insights into its divisibility. A number is divisible by another number if it can be divided by that number without leaving a remainder. In the case of 33, it is divisible by 1, 3, 11, and 33.
Relationship with Multiples
Multiples are the numbers obtained by multiplying a given number by other whole numbers. The multiples of 33 are infinite, as any multiple of 33 can be expressed by multiplying it by any positive integer. For example, some multiples of 33 include 33, 66, 99, 132, and so on.
Conclusion
In conclusion, the factors of 33 are 1, 3, 11, and 33. These factors help us understand the relationships, properties, and divisibility of the number 33. By exploring the factors of a number, we gain valuable insights into its mathematical characteristics. Factors and their properties play a significant role in various mathematical calculations and concepts.
FAQs (Frequently Asked Questions)
- Q: How many factors does 33 have? A: 33 has four factors: 1, 3, 11, and 33.
- Q: What is the prime factorization of 33?
- A: The prime factorization of 33 is 3 x 11.
- Q: Is 33 a prime number? A: No, 33 is not a prime number because it has factors other than 1 and itself.
- Q: Can 33 be divided evenly by 2? A: No, 33 is not divisible by 2 because it leaves a remainder of 1 when divided by 2.
- Q: What are the common multiples of 33 and 44? A: The common multiples of 33 and 44 are numbers that can be evenly divided by both 33 and 44. Some common multiples include 132, 264, 396, and so on.
- Q: Can 33 be divided evenly by 5? A: No, 33 is not divisible by 5 because it leaves a remainder of 3 when divided by 5.
- These frequently asked questions provide further insights into the factors and properties of 33. By understanding the factors of a number like 33, we can deepen our understanding of its mathematical characteristics and relationships.