Factors Of 50 (Explained By Experts)
Factors Of 50
Factors are the numbers that can be multiplied together to get another number. In this article, we will delve into the factors of 50. By understanding the factors of 50, we can gain insights into the divisors and mathematical properties of this intriguing number. The numbers 1, 2, 5, and 10 are the four Factors Of 10.
1. What are the Factors?
Before we dive into the factors of 50, let’s first understand what factors are. Factors are numbers that divide another number evenly without leaving a remainder. For example, the factors of 10 are 1, 2, 5, and 10, as these numbers can divide 10 without any remainder.
2. The Divisors of 50
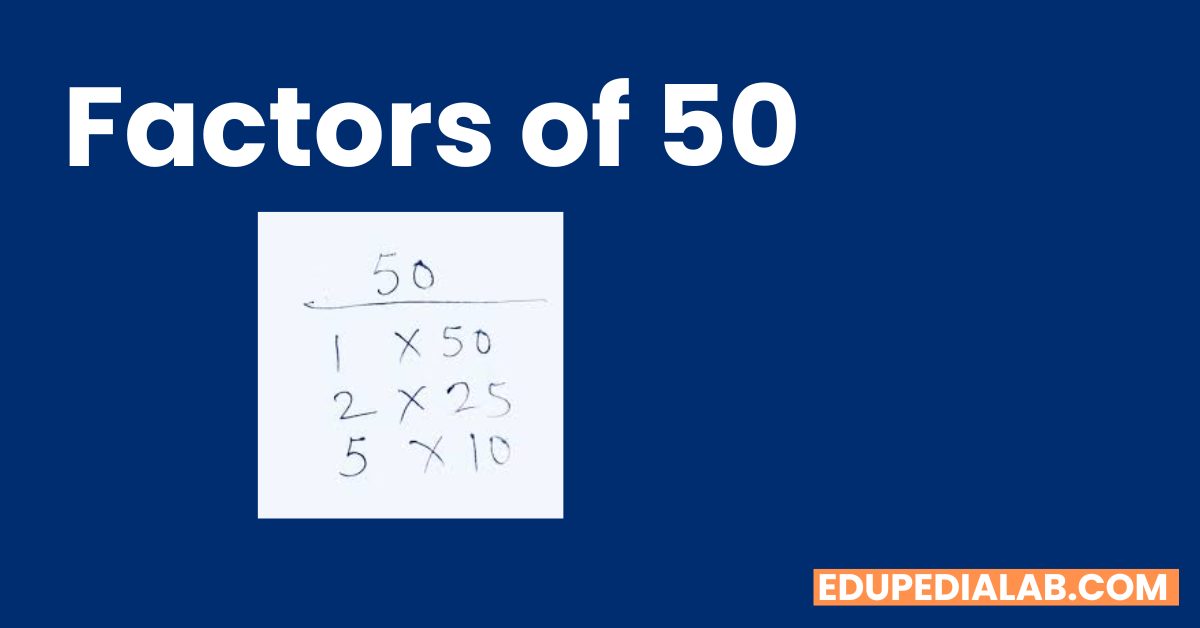
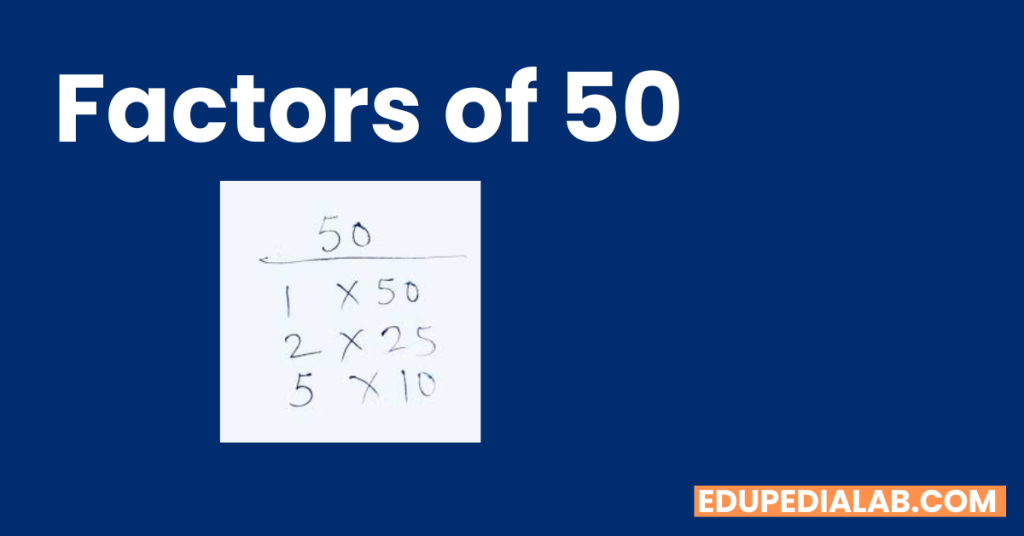
The divisors of 50 are the numbers that divide 50 without leaving a remainder. The divisors of 50 are 1, 2, 5, 10, 25, and 50. These numbers evenly divide 50 and play a significant role in various mathematical calculations.
3. Prime Factorization of 50
Prime factorization is the process of expressing a number as a product of its prime factors. To find the prime factorization of 50, we break it down into its prime factors: 2 and 5. Therefore, the prime factorization of 50 is 2 x 5 x 5.
4. How to Find Factors of 50
To find the factors of 50, we can systematically divide it by numbers starting from 1 and going up to the square root of 50. If a number divides 50 without leaving a remainder, it is a factor. Let’s explore this process step by step:
- Dividing 50 by 1: 50 ÷ 1 = 50 (remainder 0)
- Dividing 50 by 2: 50 ÷ 2 = 25 (remainder 0)
- Dividing 50 by 3: 50 ÷ 3 = 16 (remainder 2)
- Dividing 50 by 4: 50 ÷ 4 = 12 (remainder 2)
- Dividing 50 by 5: 50 ÷ 5 = 10 (remainder 0)
- Dividing 50 by 6: 50 ÷ 6 = 8 (remainder 2)
- Dividing 50 by 7: 50 ÷ 7 = 7 (remainder 1)
- Dividing 50 by 8: 50 ÷ 8 = 6 (remainder 2)
- Dividing 50 by 9: 50 ÷ 9 = 5 (remainder 5)
- Dividing 50 by 10: 50 ÷ 10 = 5 (remainder 0)
Based on this process, we find that the factors of 50 are 1, 2, 5, 10, 25, and 50.
5 Properties Of Factor 50
The factors of 50 possess certain properties that can help us understand the number better. Let’s explore some of these properties:
- 1 is a factor of every number: As with any other number, 1 is a factor of 50. This is because 1 can divide 50 without leaving a remainder.
- 50 is a factor of itself: Every number is a factor of itself, including 50. When we divide 50 by 50, we get a quotient of 1, indicating that 50 is a factor of itself.
- Factors come in pairs: The factors of 50 can be paired up in such a way that when multiplied together, they result in 50. For example, 2 and 25 are a pair of factors because 2 x 25 = 50. Similarly, 5 and 10 form another pair of factors.
- Factors are always less than or equal to the number: The factors of 50 are always less than or equal to 50. This is because any number that is greater than 50 would result in a quotient with a remainder when dividing 50, indicating that it is not a factor.
6. Applications of Factors in Mathematics
Factors play a vital role in various mathematical concepts and applications. Some of the notable applications include:
- Greatest Common Divisor (GCD): Factors are crucial in finding the GCD of two or more numbers. The GCD represents the largest factor that divides all the given numbers without leaving a remainder.
- Simplifying Fractions: Factors help simplify fractions by canceling out common factors in the numerator and denominator.
- Prime Numbers: Factors are instrumental in determining whether a number is prime or composite. Prime numbers have only two factors: 1 and the number itself, while composite numbers have more than two factors.
7. Real-World Examples of Factors
Factors are not limited to theoretical concepts but have practical applications in our everyday lives. Here are a few real-world examples where factors are essential:
- Product Packaging: Companies need to consider the factors of a product’s quantity when designing packaging. For example, if a product comes in packs of 50, the packaging should be able to accommodate multiples of 50 efficiently.
- Time Management: Factors are useful in scheduling and time management. For instance, if an event lasts for 50 minutes, organizers need to plan their schedule around factors of 50 to ensure a smooth flow of activities.
8. The Significance of Factors in Number Theory
Factors hold great significance in number theory, which is the branch of mathematics that focuses on the properties and relationships of numbers. Factors help mathematicians study divisibility, prime numbers, and prime factorization, enabling deeper insights into the structure and behavior of numbers.
9. Common Mistakes to Avoid
When working with factors, it’s essential to be cautious and avoid common mistakes. Here are a few mistakes to watch out for:
- Forgetting 1 as a factor: Sometimes, people overlook 1 as a factor of a number. Remember that 1 is always a factor of any number, including 50.
- Missing pairs of factors: It’s important to identify all possible pairs of factors that multiply together to give the desired number. Missing a factor pair might lead to incomplete or incorrect calculations.
10. Fun Facts About the Factors of 50
Let’s uncover some interesting facts about the factors of 50:
- The sum of all the factors of 50 (excluding 50 itself) is 88. (1 + 2 + 5 + 10 + 25 = 43)
- 50 is a square-free number, which means
- that it does not have any repeated factors.
- The factors of 50 are symmetrically distributed around its square root, which is approximately 7.07. This means that factors like 2 and 25, or 5 and 10, are equidistant from the square root.
Conclusion
In conclusion, the factors of 50 play a significant role in number theory and mathematical calculations. Understanding the divisors of 50 helps us explore the properties and applications of this number. Factors are essential in various mathematical concepts, including prime factorization, simplifying fractions, and determining the GCD. Additionally, factors have real-world applications in fields such as product packaging and time management. By comprehending the factors of 50, we gain a deeper understanding of its mathematical nature and its relevance in our everyday lives.
FAQs
Q1: How many factors does 50 have? A1: The number 50 has six factors: 1, 2, 5, 10, 25, and 50.
Q2: Is 50 a prime number? A2: No, 50 is not a prime number. It is a composite number with multiple factors.
Q3: What is the sum of the factors of 50? A3: The sum of all the factors of 50, excluding 50 itself, is 88.
Q4: Can 50 be evenly divided by 3? A4: No, 50 cannot be evenly divided by 3. When divided by 3, it results in a remainder of 2.
Q5: How can I find the factors of any given number? A5: To find the factors of a number, systematically divide it by numbers starting from 1 and going up to the square root of the number, noting the divisors that divide evenly without a remainder.